Section 8.2 Installment Loans
275
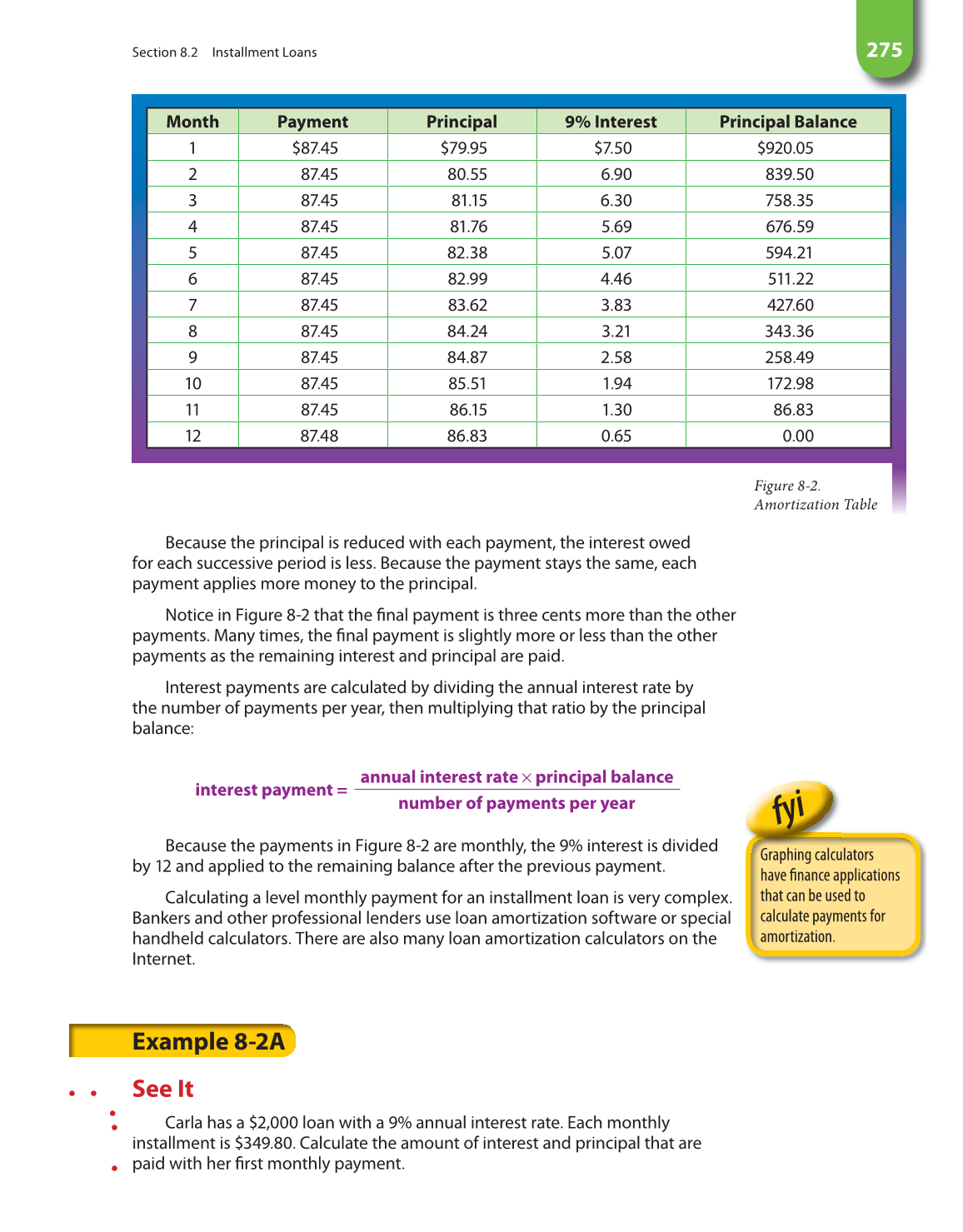
Figure 8-2.
Amortization Table
Because the principal is reduced with each payment, the interest owed
for each successive period is less. Because the payment stays the same, each
payment applies more money to the principal.
Notice in Figure 8-2 that the fi nal payment is three cents more than the other
payments. Many times, the fi nal payment is slightly more or less than the other
payments as the remaining interest and principal are paid.
Interest payments are calculated by dividing the annual interest rate by
the number of payments per year, then multiplying that ratio by the principal
balance:
interest payment =
annual interest rate × principal balance
number of payments per year
Because the payments in Figure 8-2 are monthly, the 9% interest is divided
by 12 and applied to the remaining balance after the previous payment.
Calculating a level monthly payment for an installment loan is very complex.
Bankers and other professional lenders use loan amortization software or special
handheld calculators. There are also many loan amortization calculators on the
Internet.
Example 8-2A
See It
Carla has a $2,000 loan with a 9% annual interest rate. Each monthly
installment is $349.80. Calculate the amount of interest and principal that are
paid with her fi rst monthly payment.
fyi
Graphing calculators
have fi nance applications
that can be used to
calculate payments for
amortization.
Month Payment Principal 9% Interest Principal Balance
1 $87.45 $79.95 $7.50 $920.05
2 87.45 80.55 6.90 839.50
3 87.45 81.15 6.30 758.35
4 87.45 81.76 5.69 676.59
5 87.45 82.38 5.07 594.21
6 87.45 82.99 4.46 511.22
7 87.45 83.62 3.83 427.60
8 87.45 84.24 3.21 343.36
9 87.45 84.87 2.58 258.49
10 87.45 85.51 1.94 172.98
11 87.45 86.15 1.30 86.83
12 87.48 86.83 0.65 0.00