Chapter 3 CNC Math 61
Trigonometry
Trigonometry is the area of mathematics that deals with the relationship
between the sides and angles of a triangle. Triangles are measured to find
the length of a side (leg) or to find the number of degrees in an angle. In
CNC machining, trigonometry is used to determine tool location relative
to part geometry.
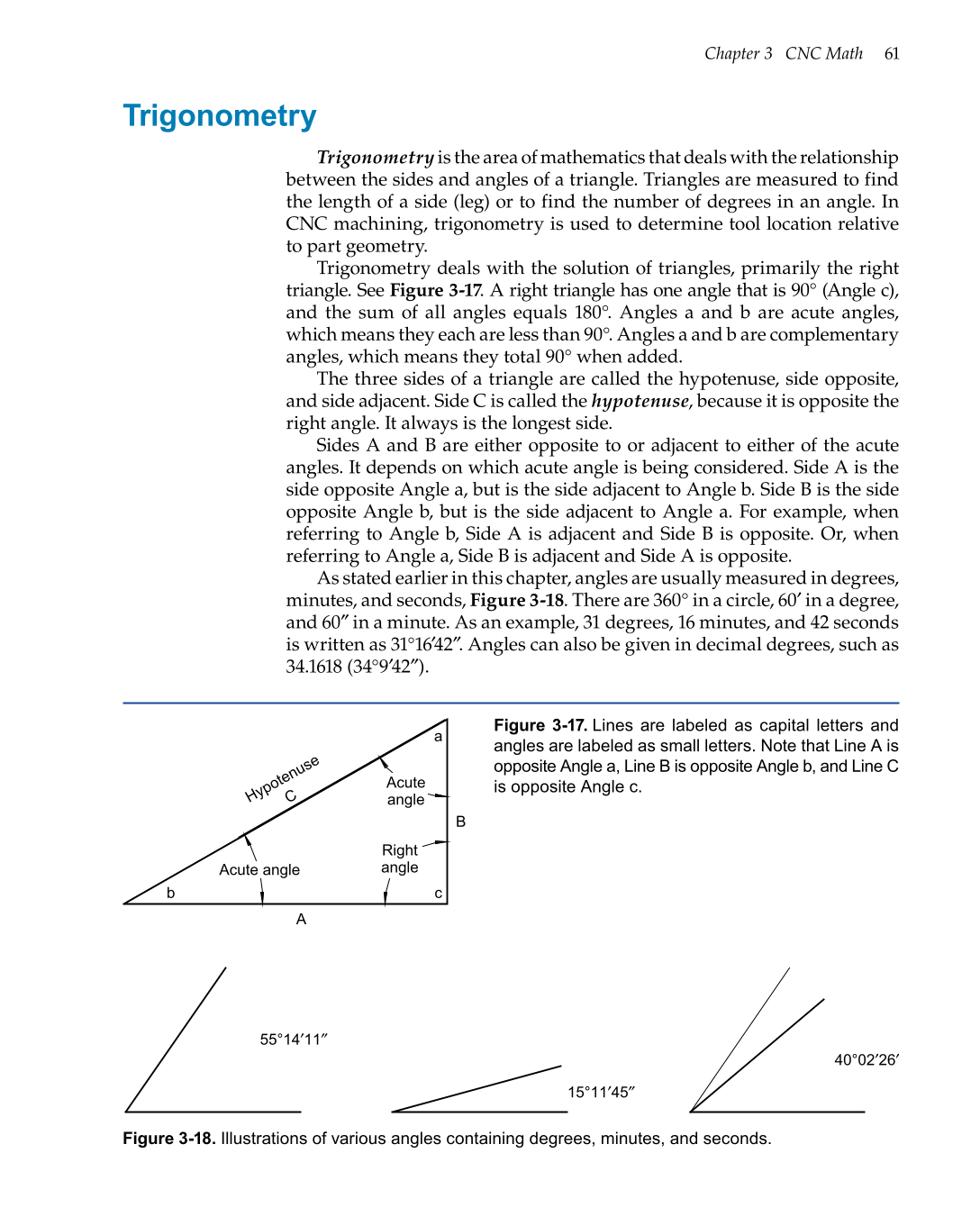
Trigonometry deals with the solution of triangles, primarily the right
triangle. See Figure 3-17. A right triangle has one angle that is 90° (Angle c),
and the sum of all angles equals 180°. Angles a and b are acute angles,
which means they each are less than 90°. Angles a and b are complementary
angles, which means they total 90° when added.
The three sides of a triangle are called the hypotenuse, side opposite,
and side adjacent. Side C is called the hypotenuse, because it is opposite the
right angle. It always is the longest side.
Sides A and B are either opposite to or adjacent to either of the acute
angles. It depends on which acute angle is being considered. Side A is the
side opposite Angle a, but is the side adjacent to Angle b. Side B is the side
opposite Angle b, but is the side adjacent to Angle a. For example, when
referring to Angle b, Side A is adjacent and Side B is opposite. Or, when
referring to Angle a, Side B is adjacent and Side A is opposite.
As stated earlier in this chapter, angles are usually measured in degrees,
minutes, and seconds, Figure 3-18. There are 360° in a circle, 60′ in a degree,
and 60″ in a minute. As an example, 31 degrees, 16 minutes, and 42 seconds
is written as 31°16′42″. Angles can also be given in decimal degrees, such as
34.1618 (34°9′42″).
Hypotenuse
Acute angle
Acute
angle
Right
angle
b
a
c
A
B
C
Figure 3-17. Lines are labeled as capital letters and
angles are labeled as small letters. Note that Line A is
opposite Angle a, Line B is opposite Angle b, and Line C
is opposite Angle c.
Figure 3-18. Illustrations of various angles containing degrees, minutes, and seconds.
40°02′26″
15°11′45″
55°14′11″