Chapter 3 CNC Math 69
Pythagorean Theorem
The Pythagorean theorem states a special relationship that exists
among the three sides of a right triangle. It states that the length of the
hypotenuse squared equals the sum of the squares of the other two side lengths. So,
if the lengths of any two sides of a right triangle are given, the length of the
third side can be calculated by using the Pythagorean theorem:
A2 + B2 = C2
In Figure 3-29, Side C is equal to 5 and Side B is equal to 3. The value for
A (the third side of the triangle) can be determined by using the formula
C2 = A2 + B2. To solve for A, substitute the known values into the formula to
get 52 = A2 + 32, then square the values to get 25 = A2 + 9. Next, isolate the
unknown variable by subtracting 9 from both sides of the equation to get
16 = A2. Finally, take the square root of both sides of the equation, to get 4 = A.
So, the length of Side A is 4.
To cut a 90° rounded corner on a workpiece, we can use the Pythagorean
theorem to plot the toolpath of the cutter. See Figure 3-30. The radius on
the workpiece is 1″. The cutter diameter is 0.25″ (0.125″ radius).
To cut partial arcs, we can use a combination of trigonometric
functions and the Pythagorean theorem to plot the positions of the cutter.
See Figure 3-31.
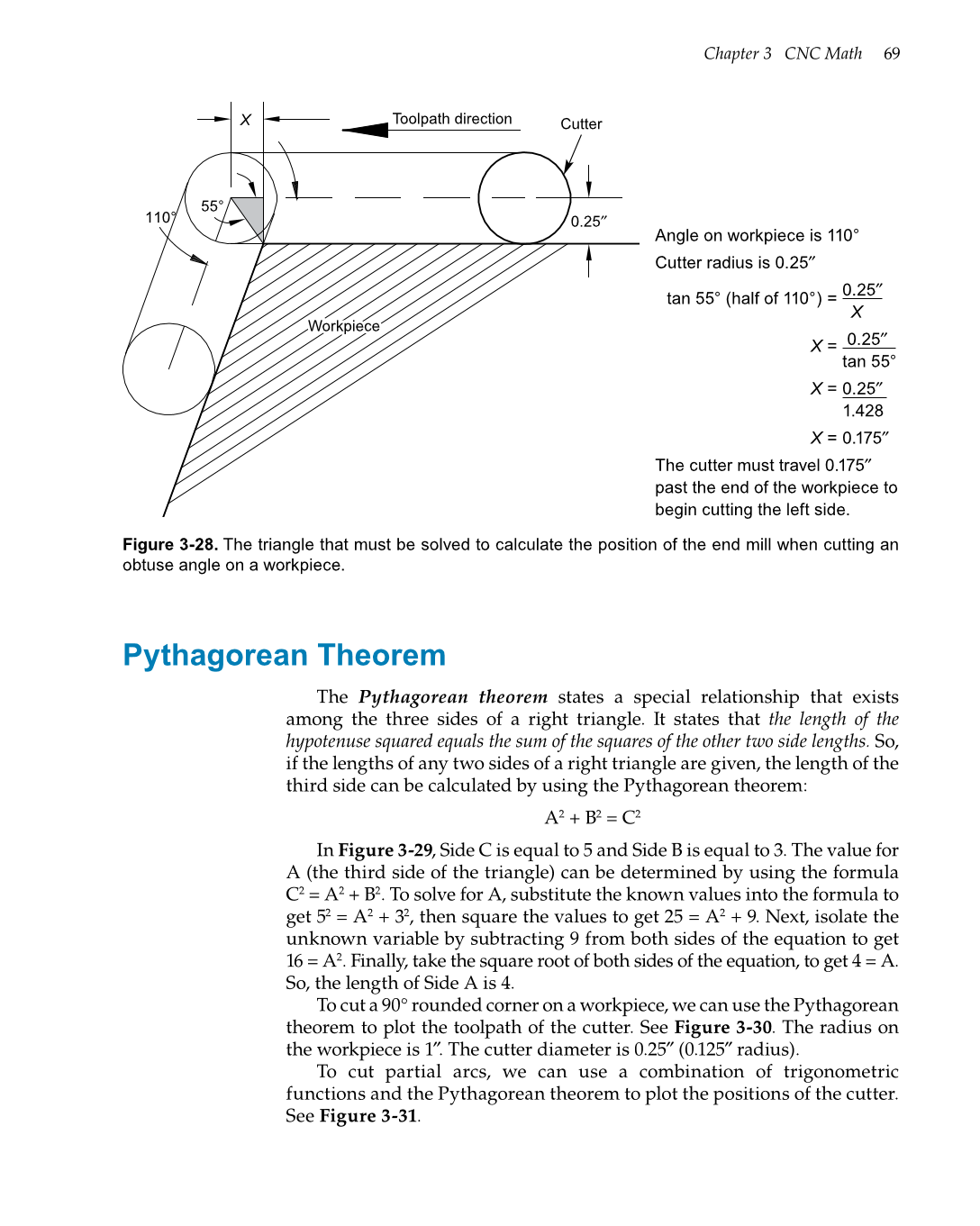
Angle on workpiece is 110°
Cutter radius is 0.25″
tan 55° (half of 110°) =
0.25″
X
X =
0.25″
tan 55°
X = 0.25″
1.428
X = 0.175″
The cutter must travel 0.175″
past the end of the workpiece to
begin cutting the left side.
X
Workpiece
Toolpath direction
Cutter
X
55°
110°
Workpiece
0.25″
Figure 3-28. The triangle that must be solved to calculate the position of the end mill when cutting an
obtuse angle on a workpiece.