178 Section 2 Drafting Techniques and Skills
3. Enter the
Circle
command and draw
a circle with a radius (R
2
) equal to the
length of the other given side. Use the
Endpoint object snap to locate the center
point of the circle at Point A. The inter-
section of the two circles is Point C.
4. Enter the
Line
command and draw
Lines AC and BC. Use the Endpoint and
Intersection object snaps. Triangle ACB is
the required right triangle.
Construct a
Right Triangle
CAD
Using a Compass
(Manual Procedure)
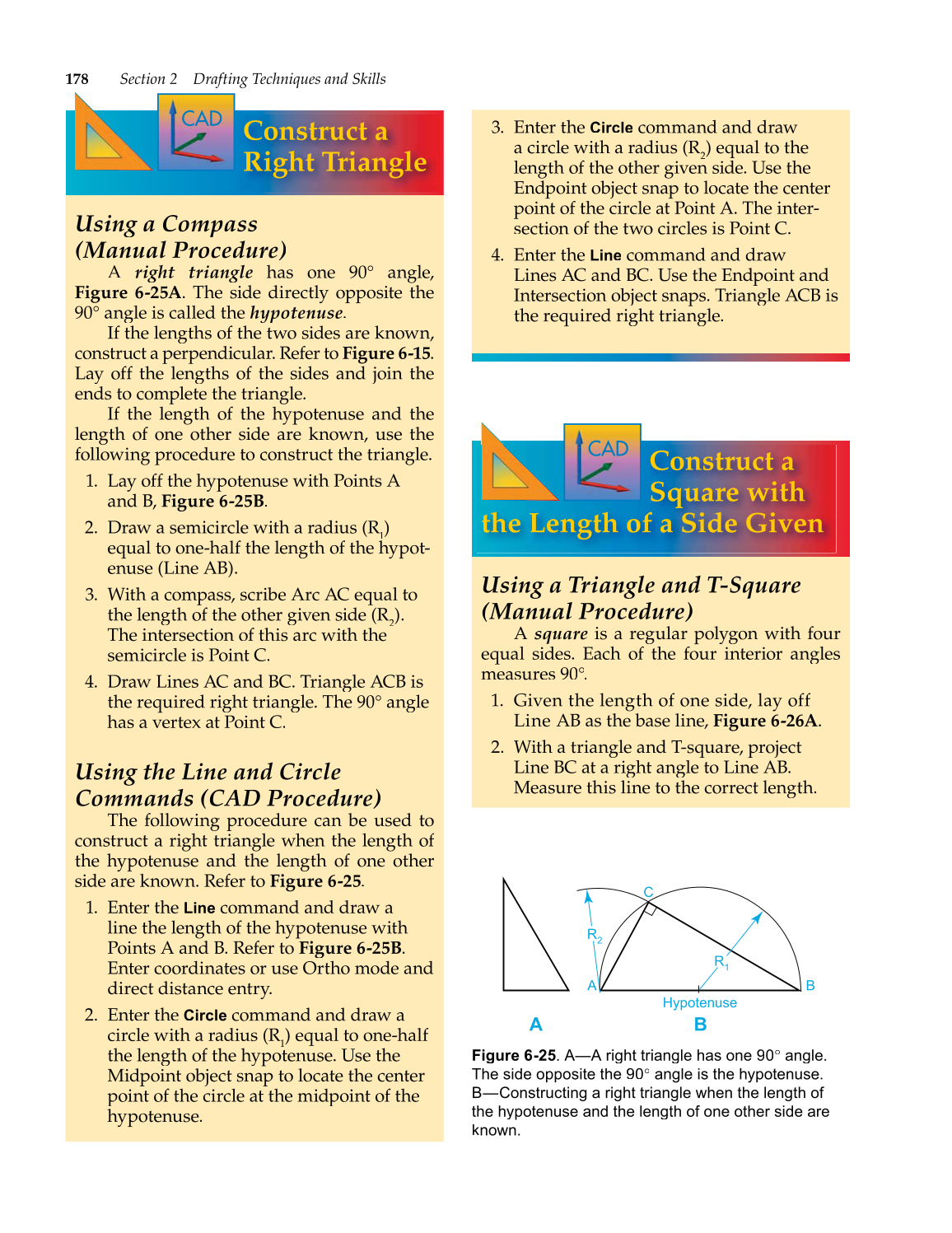
A right triangle has one 90° angle,
Figure 6-25A. The side directly opposite the
90° angle is called the hypotenuse.
If the lengths of the two sides are known,
construct a perpendicular. Refer to Figure 6-15.
Lay off the lengths of the sides and join the
ends to complete the triangle.
If the length of the hypotenuse and the
length of one other side are known, use the
following procedure to construct the triangle.
1. Lay off the hypotenuse with Points A
and B, Figure 6-25B.
2. Draw a semicircle with a radius (R
1
)
equal to one-half the length of the hypot-
enuse (Line AB).
3. With a compass, scribe Arc AC equal to
the length of the other given side (R
2
).
The intersection of this arc with the
semicircle is Point C.
4. Draw Lines AC and BC. Triangle ACB is
the required right triangle. The 90° angle
has a vertex at Point C.
Using the Line and Circle
Commands (CAD Procedure)
The following procedure can be used to
construct a right triangle when the length of
the hypotenuse and the length of one other
side are known. Refer to Figure 6-25.
1. Enter the
Line
command and draw a
line the length of the hypotenuse with
Points A and B. Refer to Figure 6-25B.
Enter coordinates or use Ortho mode and
direct distance entry.
2. Enter the
Circle
command and draw a
circle with a radius (R
1
) equal to one-half
the length of the hypotenuse. Use the
Midpoint object snap to locate the center
point of the circle at the midpoint of the
hypotenuse.
Figure 6-25. A—A right triangle has one 90° angle.
The side opposite the 90° angle is the hypotenuse.
B—Constructing a right triangle when the length of
the hypotenuse and the length of one other side are
known.
R2
R1
A
Hypotenuse
B
C
A B
Using a Triangle and T-Square
(Manual Procedure)
A square is a regular polygon with four
equal sides. Each of the four interior angles
measures 90°.
1. Given the length of one side, lay off
Line AB as the base line, Figure 6-26A.
2. With a triangle and T-square, project
Line BC at a right angle to Line AB.
Measure this line to the correct length.
Construct a
Square with
the Length of a Side Given
CAD