Chapter 6 Basic Geometric Constructions 187
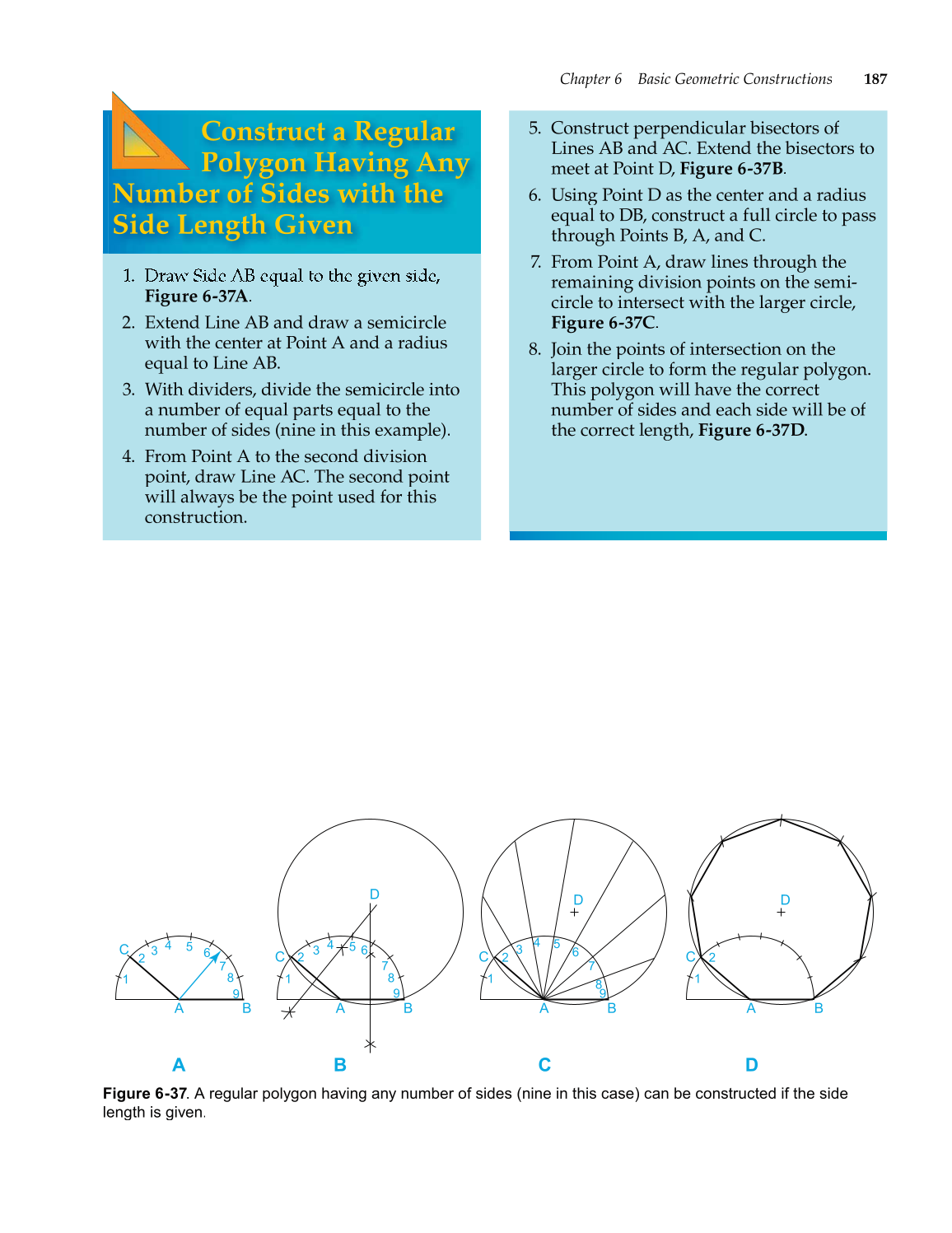
5. Construct perpendicular bisectors of
Lines AB and AC. Extend the bisectors to
meet at Point D, Figure 6-37B.
6. Using Point D as the center and a radius
equal to DB, construct a full circle to pass
through Points B, A, and C.
7. From Point A, draw lines through the
remaining division points on the semi-
circle to intersect with the larger circle,
Figure 6-37C.
8. Join the points of intersection on the
larger circle to form the regular polygon.
This polygon will have the correct
number of sides and each side will be of
the correct length, Figure 6-37D.
Figure 6-37. A regular polygon having any number of sides (nine in this case) can be constructed if the side
length is given.
A B
C
1
2
3
4
5
6
7
8
9
A
A B
C
D
1
2
3
4
5
6
7
8
9
B
A B
C
D
1
2
3
4
5
6
7
8
9
C
A B
C
D
1
2
D
Figure 6-37A.
2. Extend Line AB and draw a semicircle
with the center at Point A and a radius
equal to Line AB.
3. With dividers, divide the semicircle into
a number of equal parts equal to the
number of sides (nine in this example).
4. From Point A to the second division
point, draw Line AC. The second point
will always be the point used for this
construction.
Construct a Regular
Polygon Having Any
Number of Sides with the
Side Length Given