222
Electricity and Basic Electronics
next section dealing with transformers. The
formula for mutual inductance is
M = K √L1
_____
L
2
(12-3)
where
M = Mutual inductance.
K = Coeffi cient of coupling (percent
of coupling).
L
1
= Inductance of coil 1.
L
2
= Inductance of coil 2.
Suppose we have two coils placed near
each other. One has an inductance of 12.5 H,
and the other has an inductance of 2 H. You
are given a coeffi cient of coupling of 50%.
Alternating current is passed through coil 1,
creating lines of force. When this occurs, 50%
of these lines of force cut across coil 2. We can
use the formula to fi nd the mutual inductance
between the two coils.
M = K √L1L2
____
We know that L
1
= 12.5 H and L
2
= 2 H. We
also know that K = 0.5 or 50%. Substituting
these values into the mutual induction
formula, we have
M = 0.5 √12.5
____________
H × 2 H
= 0.5 √25
_____
H
= 0.5 × 5 H
= 2.5 H
These two coils have a mutual inductance
of 2.5 H.
Example 12-3:
Suppose two coils of wire have an induc-
tance of 20 mH and 5 mH, and their coeffi -
cient of coupling is 75%. What is the mutual
inductance of the two coils? Begin with the
formula for mutual inductance:
M = K √L1L2
____
We know the values of L
1
, L
2
, and K. Substi-
tute these values into the formula.
Mutual Inductance
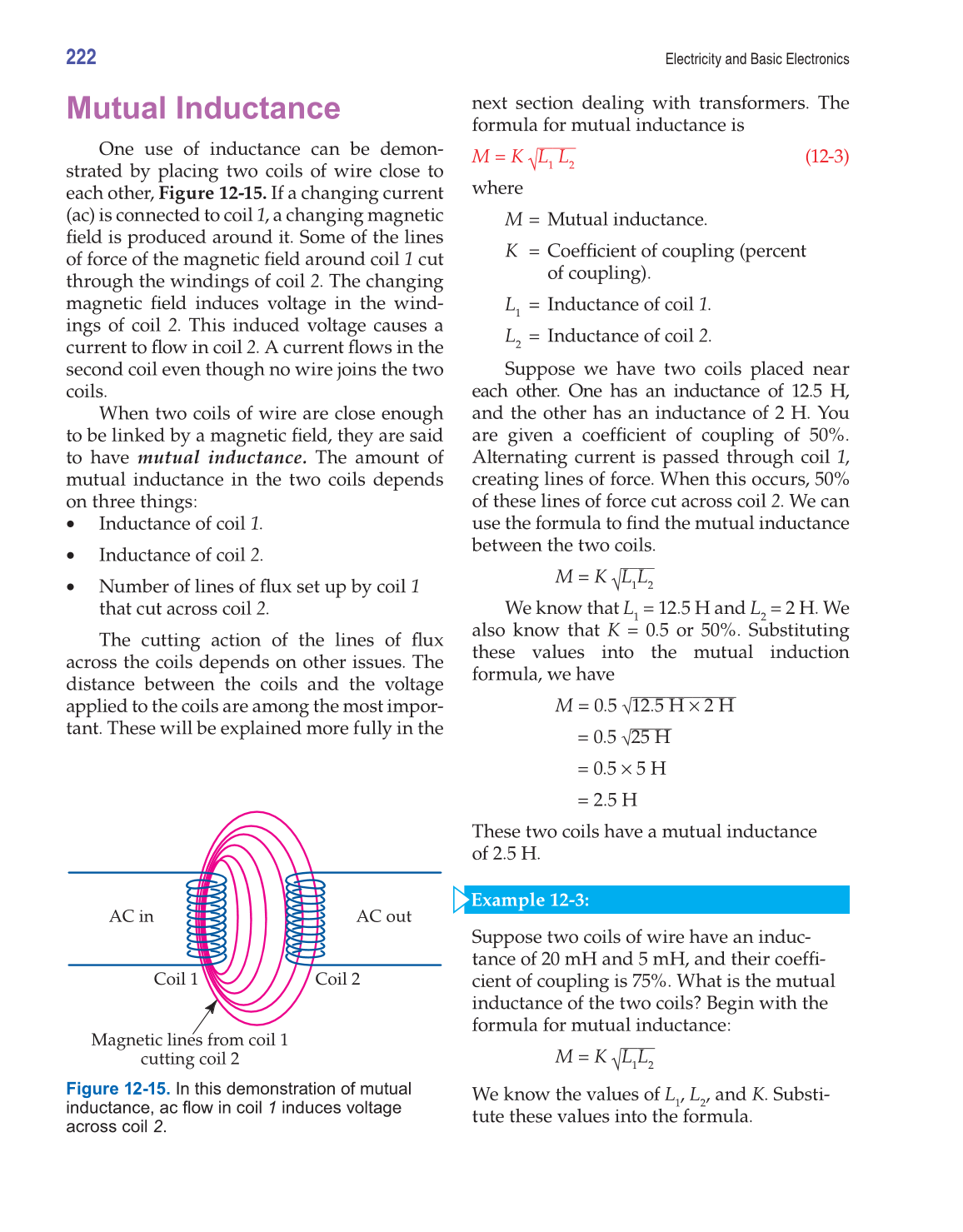
One use of inductance can be demon-
strated by placing two coils of wire close to
each other, Figure 12-15. If a changing current
(ac) is connected to coil 1, a changing magnetic
fi eld is produced around it. Some of the lines
of force of the magnetic fi eld around coil 1 cut
through the windings of coil 2. The changing
magnetic fi eld induces voltage in the wind-
ings of coil 2. This induced voltage causes a
current to fl ow in coil 2. A current fl ows in the
second coil even though no wire joins the two
coils.
When two coils of wire are close enough
to be linked by a magnetic fi eld, they are said
to have mutual inductance. The amount of
mutual inductance in the two coils depends
on three things:
• Inductance of coil 1.
• Inductance of coil 2.
• Number of lines of fl ux set up by coil 1
that cut across coil 2.
The cutting action of the lines of fl ux
across the coils depends on other issues. The
distance between the coils and the voltage
applied to the coils are among the most impor-
tant. These will be explained more fully in the
Figure 12-15. In this demonstration of mutual
inductance, ac fl ow in coil 1 induces voltage
across coil 2.
AC out AC in
Coil 1 Coil 2
Magnetic lines from coil 1
cutting coil 2