Copyright Goodheart-Willcox Co., Inc.
Chapter 11 Electrical Engineering 229
Voltage in a series circuit is equal to the
sum of the voltage drop across each load in the
circuit. The voltage drop across each load varies
in proportion to its resistance, but the sum of all
drops equals the applied voltage. Use a string of
60 holiday lights wired in series as an example.
They would be plugged into a common 120 V
household electric outlet. To fi nd the voltage drop
across each light, divide 120 V by 60 lights. There
is a voltage drop of 2 V across each light.
This can be expressed as:
Et = E1 + E2 + E3 +…+ EN
Total resistance in a series circuit is equal
to the sum of the resistance of each individual
load. For example, picture three resistors wired
in series. Their values are 10 Ω, 20 Ω, and 30 Ω.
The total resistance in the circuit is the sum of all
resistances, or 60 Ω. This can be expressed as:
Rt = R1 + R2 + R3 +…+ RN
Current is constant throughout a series circuit.
Think of water fl owing through a garden hose.
Whatever fl ow goes into one end of the hose will
fl ow through and come out the other end. This is the
same in electricity. In the previous example, the three
resistors have a total resistance of 60 Ω. If they are
connected to a 120 V power source, there will be 2 A
(120 V / 60 Ω = 2 A) of current fl owing at every single
point in the circuit. No matter where the current is
tested, it would read 2 A. This can be expressed as:
It = I1 = I2 = I3 =…= IN
Parallel Circuits
Parallel circuits have more than one load and
have multiple paths for current fl ow. Each path is
a branch and contains one load. Current is divided
between the branches in proportion to the resis-
tance of the load in each branch. In other words,
the branch with the lowest resistance will have the
highest current fl ow. The voltage across the load in
each branch is equal to the source voltage.
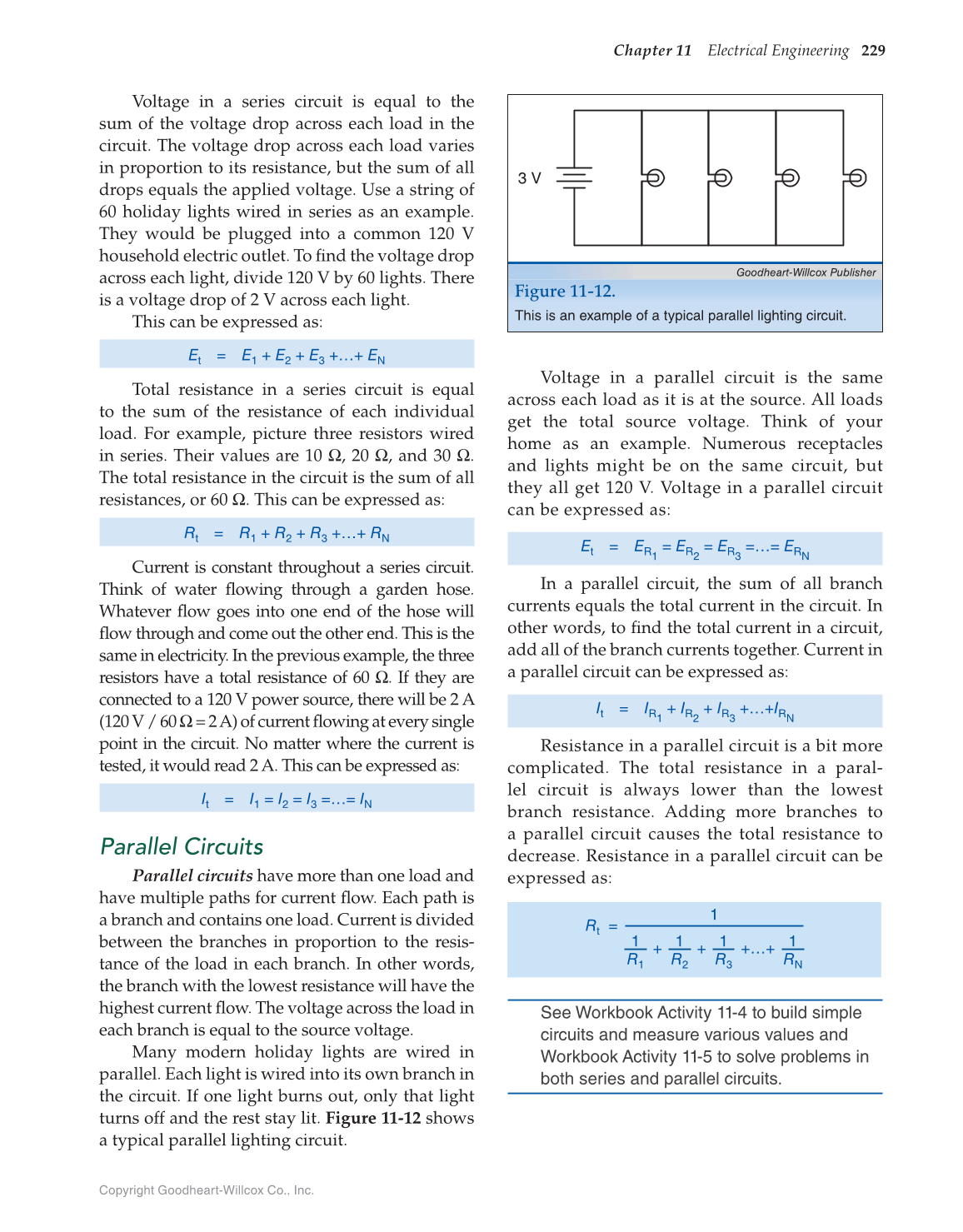
Many modern holiday lights are wired in
parallel. Each light is wired into its own branch in
the circuit. If one light burns out, only that light
turns off and the rest stay lit. Figure 11-12 shows
a typical parallel lighting circuit.
Voltage in a parallel circuit is the same
across each load as it is at the source. All loads
get the total source voltage. Think of your
home as an example. Numerous receptacles
and lights might be on the same circuit, but
they all get 120 V. Voltage in a parallel circuit
can be expressed as:
Et = ER1 = ER2 = ER3 =…= ERN
In a parallel circuit, the sum of all branch
currents equals the total current in the circuit. In
other words, to fi nd the total current in a circuit,
add all of the branch currents together. Current in
a parallel circuit can be expressed as:
It = IR1 + IR2 + IR3 +…+IRN
Resistance in a parallel circuit is a bit more
complicated. The total resistance in a paral-
lel circuit is always lower than the lowest
branch resistance. Adding more branches to
a parallel circuit causes the total resistance to
decrease. Resistance in a parallel circuit can be
expressed as:
Rt =
1
1
+
1
+
1
+…+
1
R1 R2 R3 RN
See Workbook Activity 11-4 to build simple
circuits and measure various values and
Workbook Activity 11-5 to solve problems in
both series and parallel circuits.
3 V
Goodheart-Willcox Publisher
Figure 11-12. 
This is an example of a typical parallel lighting circuit.