194 GD&T: Application and Interpretation
Copyright Goodheart-Willcox Co., Inc.
One signifi cant reason for not placing datum
feature symbols on centerlines is that the standard
specifi cally says not to do it. There is no defi ned
meaning for a datum feature symbol placed on
a centerline. The absence of a defi ned meaning
should be adequate for not placing datum feature
symbols on centerlines.
Another reason for prohibiting the placement
of datum feature symbols on centerlines is the
confusion it can cause. Consider the placement of
a datum feature symbol on a centerline that passes
through three holes. See Figure 6-53. The fi gure
shows a centerline through three holes, and it is
identifi ed as datum A. Because the datum feature
symbol is on the centerline, it is not known which
holes to use for the location of datum plane A.
Should all three holes be used, or should only two
be selected? If two are used, which two?
It is important to realize that three holes may
not be produced perfectly on one line. When the
produced holes are not in a straight line, two of
them must be selected to establish the datum. The
given fi gure shows the effect of arbitrarily select-
ing two holes to establish datum A. (Selection must
be arbitrary because the drawing does not indicate
which holes to use.) Selecting two holes at a time
results in three possible datums. Which pair of
holes should be used? This question does not have
an answer because the datum feature symbol is
not properly applied. One correct method for iden-
tifying datums that pass through multiple holes is
shown in Figure 6-52.
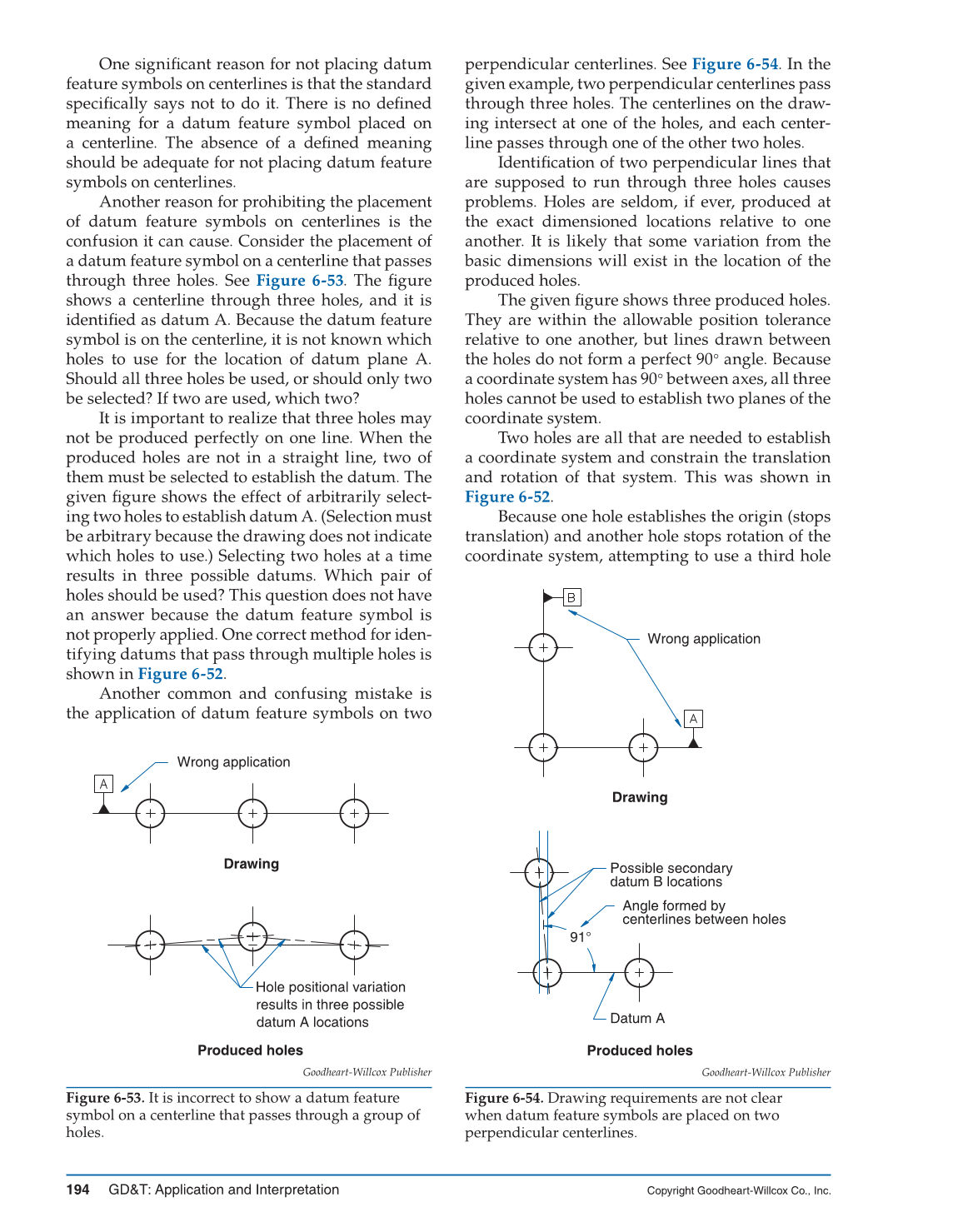
Another common and confusing mistake is
the application of datum feature symbols on two
perpendicular centerlines. See Figure 6-54. In the
given example, two perpendicular centerlines pass
through three holes. The centerlines on the draw-
ing intersect at one of the holes, and each center-
line passes through one of the other two holes.
Identifi cation of two perpendicular lines that
are supposed to run through three holes causes
problems. Holes are seldom, if ever, produced at
the exact dimensioned locations relative to one
another. It is likely that some variation from the
basic dimensions will exist in the location of the
produced holes.
The given fi gure shows three produced holes.
They are within the allowable position tolerance
relative to one another, but lines drawn between
the holes do not form a perfect 90° angle. Because
a coordinate system has 90° between axes, all three
holes cannot be used to establish two planes of the
coordinate system.
Two holes are all that are needed to establish
a coordinate system and constrain the translation
and rotation of that system. This was shown in
Figure 6-52.
Because one hole establishes the origin (stops
translation) and another hole stops rotation of the
coordinate system, attempting to use a third hole
Hole positional variation
results in three possible
datum A locations
Drawing
Produced holes
Wrong application
Goodheart-Willcox Publisher
Figure 6-53. It is incorrect to show a datum feature
symbol on a centerline that passes through a group of
holes.
Wrong application
Drawing
Produced holes
91°
Possible secondary
datum B locations
Datum A
Angle formed by
centerlines between holes
Goodheart-Willcox Publisher
Figure 6-54. Drawing requirements are not clear
when datum feature symbols are placed on two
perpendicular centerlines.