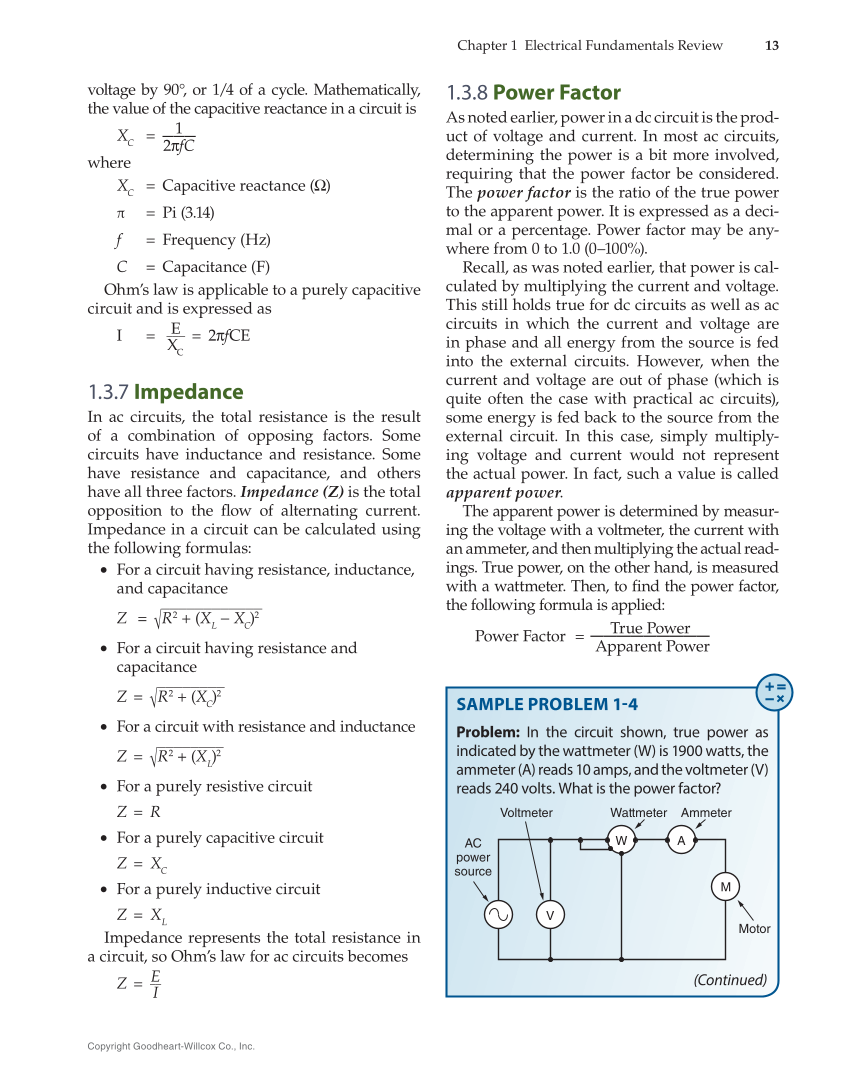
Chapter 1 Electrical Fundamentals Review 13 Copyright Goodheart-Willcox Co., Inc. voltage by 90°, or 1/4 of a cycle. Mathematically, the value of the capacitive reactance in a circuit is X C = 1 ___ 2πfC where X C = Capacitive reactance (Ω) π = Pi (3.14) f = Frequency (Hz) C = Capacitance (F) Ohm’s law is applicable to a purely capacitive circuit and is expressed as I = E ___ X C = 2πfCE 1.3.7 Impedance In ac circuits, the total resistance is the result of a combination of opposing factors. Some circuits have inductance and resistance. Some have resistance and capacitance, and others have all three factors. Impedance (Z) is the total opposition to the fl ow of alternating current. Impedance in a circuit can be calculated using the following formulas: • For a circuit having resistance, inductance, and capacitance Z = √ ____________ R2 + (XL − XC)2 • For a circuit having resistance and capacitance Z = √ ________ R2 + (X C )2 • For a circuit with resistance and inductance Z = √ ________ R2 + (X L )2 • For a purely resistive circuit Z = R • For a purely capacitive circuit Z = X C • For a purely inductive circuit Z = X L Impedance represents the total resistance in a circuit, so Ohm’s law for ac circuits becomes Z = E __ I 1.3.8 Power Factor As noted earlier, power in a dc circuit is the prod- uct of voltage and current. In most ac circuits, determining the power is a bit more involved, requiring that the power factor be considered. The power factor is the ratio of the true power to the apparent power. It is expressed as a deci- mal or a percentage. Power factor may be any- where from 0 to 1.0 (0–100%). Recall, as was noted earlier, that power is cal- culated by multiplying the current and voltage. This still holds true for dc circuits as well as ac circuits in which the current and voltage are in phase and all energy from the source is fed into the external circuits. However, when the current and voltage are out of phase (which is quite often the case with practical ac circuits), some energy is fed back to the source from the external circuit. In this case, simply multiply- ing voltage and current would not represent the actual power. In fact, such a value is called apparent power. The apparent power is determined by measur- ing the voltage with a voltmeter, the current with an ammeter, and then multiplying the actual read- ings. True power, on the other hand, is measured with a wattmeter. Then, to fi nd the power factor, the following formula is applied: Power Factor = True Power _________ Apparent Power SAMPLE PROBLEM 1-4 Problem: In the circuit shown, true power as indicated by the wattmeter (W) is 1900 watts, the ammeter (A) reads 10 amps, and the voltmeter (V) reads 240 volts. What is the power factor? M A W V Motor Ammeter Wattmeter Voltmeter AC power source (Continued)