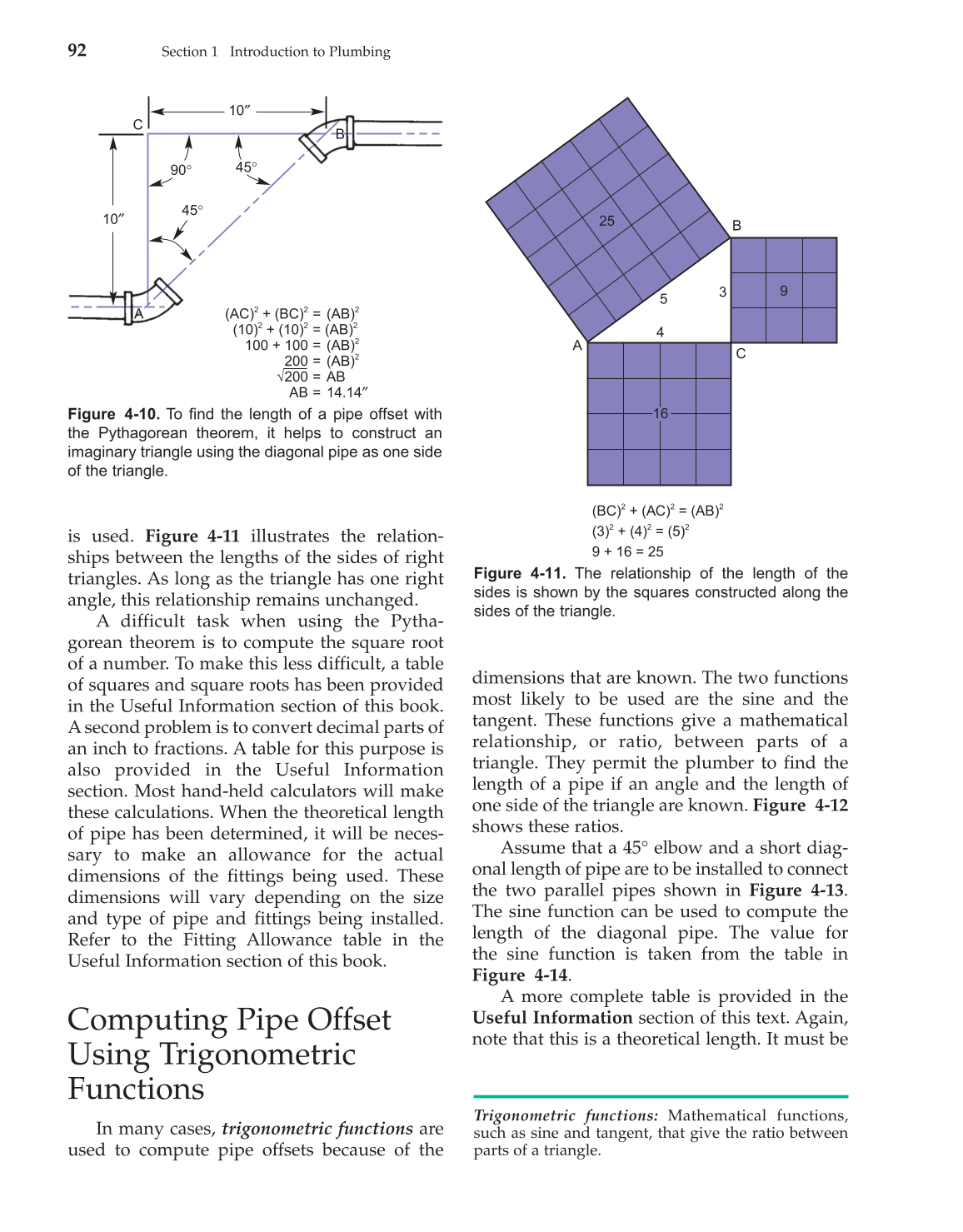
is used. Figure 4-11 illustrates the relation-
ships between the lengths of the sides of right
triangles. As long as the triangle has one right
angle, this relationship remains unchanged.
A difficult task when using the Pytha-
gorean theorem is to compute the square root
of a number. To make this less difficult, a table
of squares and square roots has been provided
in the Useful Information section of this book.
A second problem is to convert decimal parts of
an inch to fractions. A table for this purpose is
also provided in the Useful Information
section. Most hand-held calculators will make
these calculations. When the theoretical length
of pipe has been determined, it will be neces-
sary to make an allowance for the actual
dimensions of the fittings being used. These
dimensions will vary depending on the size
and type of pipe and fittings being installed.
Refer to the Fitting Allowance table in the
Useful Information section of this book.
Computing Pipe Offset
Using Trigonometric
Functions
In many cases, trigonometric functions are
used to compute pipe offsets because of the
dimensions that are known. The two functions
most likely to be used are the sine and the
tangent. These functions give a mathematical
relationship, or ratio, between parts of a
triangle. They permit the plumber to find the
length of a pipe if an angle and the length of
one side of the triangle are known. Figure 4-12
shows these ratios.
Assume that a 45° elbow and a short diag-
onal length of pipe are to be installed to connect
the two parallel pipes shown in Figure 4-13.
The sine function can be used to compute the
length of the diagonal pipe. The value for
the sine function is taken from the table in
Figure 4-14.
A more complete table is provided in the
Useful Information section of this text. Again,
note that this is a theoretical length. It must be
92
Section 1 Introduction to Plumbing
Figure 4-10. To find the length of a pipe offset with
the Pythagorean theorem, it helps to construct an
imaginary triangle using the diagonal pipe as one side
of the triangle.
A (AC)2 + (BC)2 = (AB)2
(10)2 + (10)2 = (AB)2
100 + 100 = (AB)2
200 = (AB)2
√200 = AB
AB = 14.14″
B
10″
90°
45°
45°
10″
C
Figure 4-11. The relationship of the length of the
sides is shown by the squares constructed along the
sides of the triangle.
25
5
4
3
A
B
9
C
16
(BC)2 + (AC)2 = (AB)2
(3)2 + (4)2 = (5)2
9 + 16 = 25
Trigonometric functions: Mathematical functions,
such as sine and tangent, that give the ratio between
parts of a triangle.