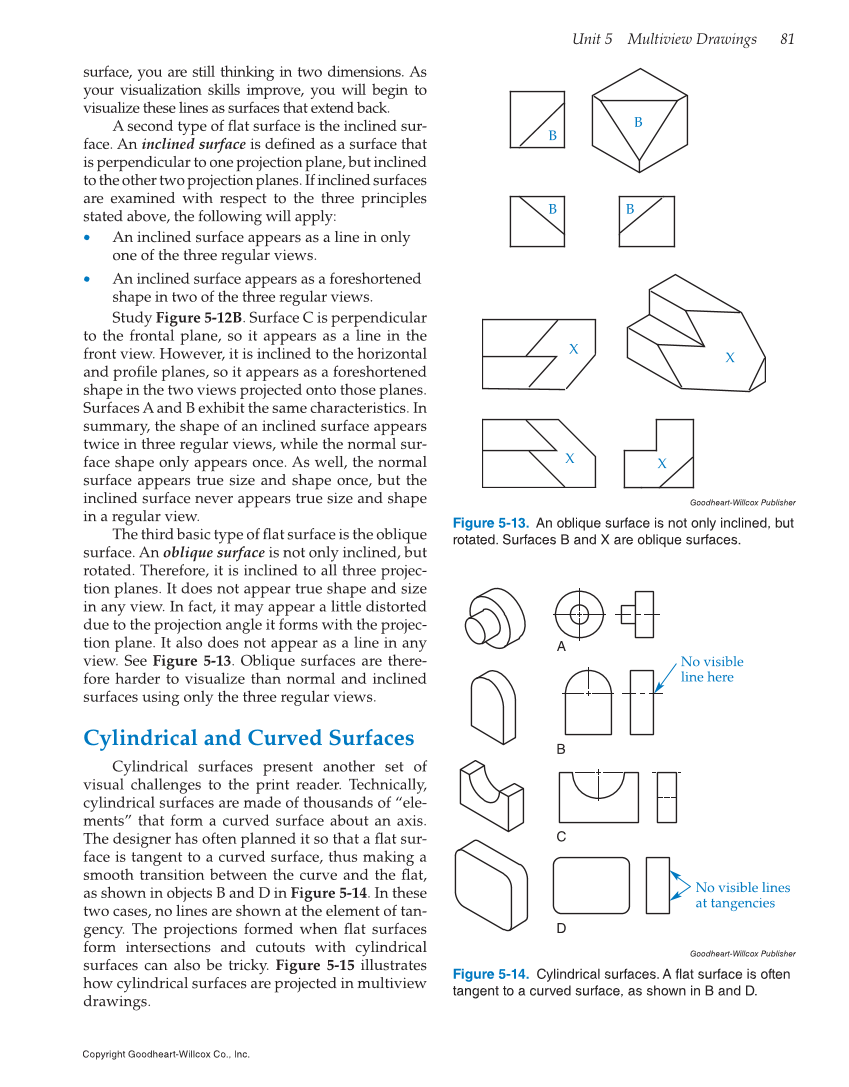
Unit 5 Multiview Drawings 81 Copyright Goodheart-Willcox Co., Inc. surface, you are still thinking in two dimensions. As your visualization skills improve, you will begin to visualize these lines as surfaces that extend back. A second type of fl at surface is the inclined sur- face. An inclined surface is defi ned as a surface that is perpendicular to one projection plane, but inclined to the other two projection planes. If inclined surfaces are examined with respect to the three principles stated above, the following will apply: • An inclined surface appears as a line in only one of the three regular views. • An inclined surface appears as a foreshortened shape in two of the three regular views. Study Figure 5-12B. Surface C is perpendicular to the frontal plane, so it appears as a line in the front view. However, it is inclined to the horizontal and profi le planes, so it appears as a foreshortened shape in the two views projected onto those planes. Surfaces A and B exhibit the same characteristics. In summary, the shape of an inclined surface appears twice in three regular views, while the normal sur- face shape only appears once. As well, the normal surface appears true size and shape once, but the inclined surface never appears true size and shape in a regular view. The third basic type of fl at surface is the oblique surface. An oblique surface is not only inclined, but rotated. Therefore, it is inclined to all three projec- tion planes. It does not appear true shape and size in any view. In fact, it may appear a little distorted due to the projection angle it forms with the projec- tion plane. It also does not appear as a line in any view. See Figure 5-13. Oblique surfaces are there- fore harder to visualize than normal and inclined surfaces using only the three regular views. Cylindrical and Curved Surfaces Cylindrical surfaces present another set of visual challenges to the print reader. Technically, cylindrical surfaces are made of thousands of “ele- ments” that form a curved surface about an axis. The designer has often planned it so that a fl at sur- face is tangent to a curved surface, thus making a smooth transition between the curve and the fl at, as shown in objects B and D in Figure 5-14. In these two cases, no lines are shown at the element of tan- gency. The projections formed when fl at surfaces form intersections and cutouts with cylindrical surfaces can also be tricky. Figure 5-15 illustrates how cylindrical surfaces are projected in multiview drawings. Goodheart-Willcox Publisher Figure 5-13. An oblique surface is not only inclined, but rotated. Surfaces B and X are oblique surfaces. B B B B X X X X Goodheart-Willcox Publisher Figure 5-14. Cylindrical surfaces. A flat surface is often tangent to a curved surface, as shown in B and D. No visible line here No visible lines at tangencies A B C D