66 CNC Machining
The six functions given are related to Angle a, but also can be applied
to Angle b as well. Therefore, Sin b = B/C, Cos b = A/C, etc., shows that
any function of Angle a is equal to the cofunction of Angle b. From that
relationship, the following are derived:
• sin a = A/C = cos b
• cos a = B/C = sin b
• tan a = A/B = cot b
• cot a = B/A = tan b
• sec a = C/B = csc b
• csc a = C/A = sec b
With Angle a and Angle b being complementary, the function of any
angle is equal to the cofunction of its complementary angle. Therefore, sin
70° = cos 20°, and tan 60° = cot 30°.
Working with Triangles
In programming, an individual will be working with various
applications of radii, such as cutter radius, arc radius, circle radius, and
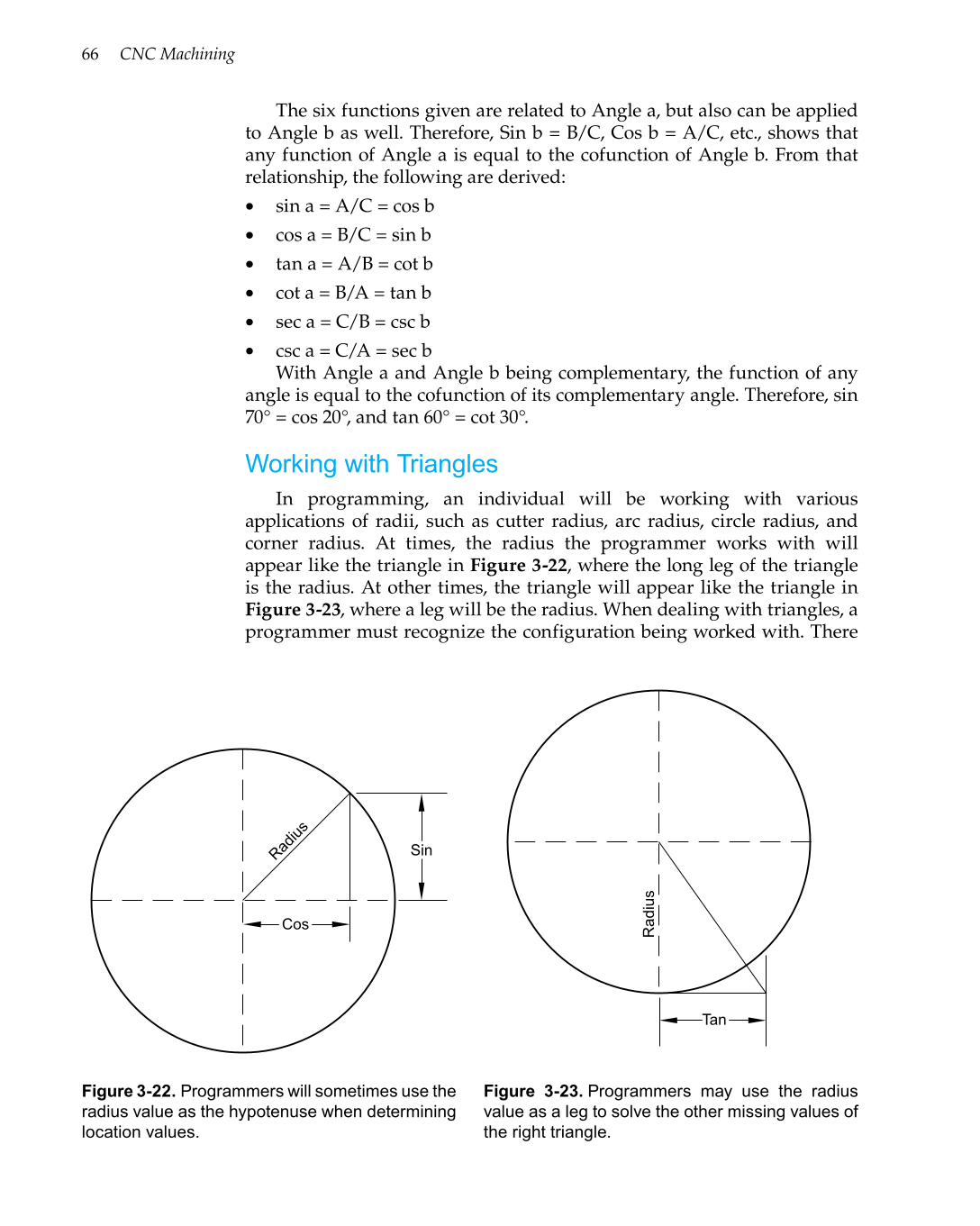
corner radius. At times, the radius the programmer works with will
appear like the triangle in Figure 3-22, where the long leg of the triangle
is the radius. At other times, the triangle will appear like the triangle in
Figure 3-23, where a leg will be the radius. When dealing with triangles, a
programmer must recognize the configuration being worked with. There
Radius
Cos
Sin
Cos
Sin
Figure 3-22. Programmers will sometimes use the
radius value as the hypotenuse when determining
location values.
Tan
Sin
Tan
Radius
Figure 3-23. Programmers may use the radius
value as a leg to solve the other missing values of
the right triangle.