Chapter 3 CNC Math 67
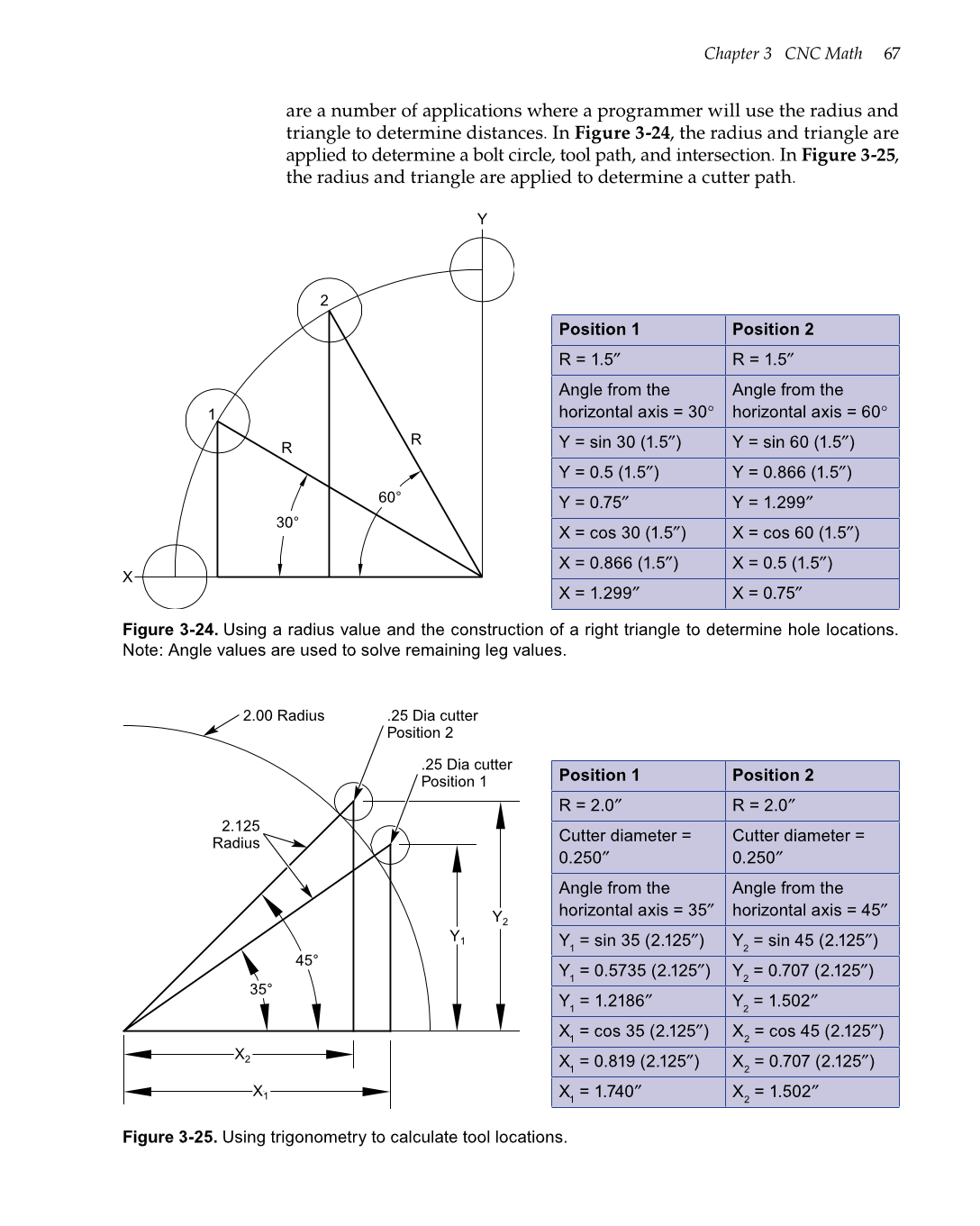
are a number of applications where a programmer will use the radius and
triangle to determine distances. In Figure 3-24, the radius and triangle are
applied to determine a bolt circle, tool path, and intersection. In Figure 3-25,
the radius and triangle are applied to determine a cutter path.
2
1
30°
60°
R
R
Y
X
Figure 3-24. Using a radius value and the construction of a right triangle to determine hole locations.
Note: Angle values are used to solve remaining leg values.
Figure 3-25. Using trigonometry to calculate tool locations.
35°
45°
Y1
Y2
X2
X1
35°
45°
Y
1
Y
2
X
2
X
1
.25 Dia cutter
Position 1
2.125
Radius
.25 Dia cutter
Position 2
2.00 Radius
Position 1 Position 2
R = 1.5″ R = 1.5″
Angle from the
horizontal axis = 30°
Angle from the
horizontal axis = 60°
Y = sin 30 (1.5″) Y = sin 60 (1.5″)
Y = 0.5 (1.5″) Y = 0.866 (1.5″)
Y = 0.75″ Y = 1.299″
X = cos 30 (1.5″) X = cos 60 (1.5″)
X = 0.866 (1.5″) X = 0.5 (1.5″)
X = 1.299″ X = 0.75″
Position 1 Position 2
R = 2.0″ R = 2.0″
Cutter diameter =
0.250″
Cutter diameter =
0.250″
Angle from the
horizontal axis = 35″
Angle from the
horizontal axis = 45″
Y1 = sin 35 (2.125″) Y2 = sin 45 (2.125″)
Y1 = 0.5735 (2.125″) Y2 = 0.707 (2.125″)
Y1 = 1.2186″ Y2 = 1.502″
X
1
= cos 35 (2.125″) X
2
= cos 45 (2.125″)
X1 = 0.819 (2.125″) X2 = 0.707 (2.125″)
X1 = 1.740″ X2 = 1.502″