Find the least common denominator of
the fraction, which is 400.
RT =
RT
=
now,
1 ÷ is the same as
1 × = = 57.1 Ω
RT
= 57.1 Ω
Another technique is to simply divide
each fraction into one, add the values, then
divide into one again.
RT
=
RT
=
RT
=
RT = 57.1 Ω
Remember to be careful when adding
decimal numbers. All decimal points must
line up to be added together properly:
0.01
0.005
+ 0.0025
Sum = 0.0175
Note: In all problems dealing with
resistance in parallel circuits, the total
resistance must always be less than the
value of any resistor in the parallel cir-
cuit. Recall that parallel circuits provide
additional pathways for current flow.
Total resistance always decreases the
more parallel resistances are added to the
circuit. Use this information to check
your work.
Equivalent Resistance
The flow of electricity in a circuit
depends upon resistance of the circuit.
This resistance can be a single resistor or
several resistors connected in series or
parallel. Regardless of how many resistors
there are or how they are connected, they
will combine together to give a total
resistance in the circuit. The total resis-
tance is the limiting factor affecting the
current. In other words, the total of all
resistances might be represented by one
resistor value. This resistance is called the
equivalent resistance of the circuit. See
Figure 7-7.
Step I. Combine R2
and R3
RT (R2 and R3) =
RT
= = 80 Ω
The circuit will appear as shown in
Figure 7-8.
40,000 Ω
500 Ω
400 Ω × 100 Ω
400 Ω + 100 Ω
1
0.0175 S
1
0.01 S + 0.005 S + 0.0025 S
1
400
7
400
7
7
400
1
1
Chapter 7 Parallel Circuits
59
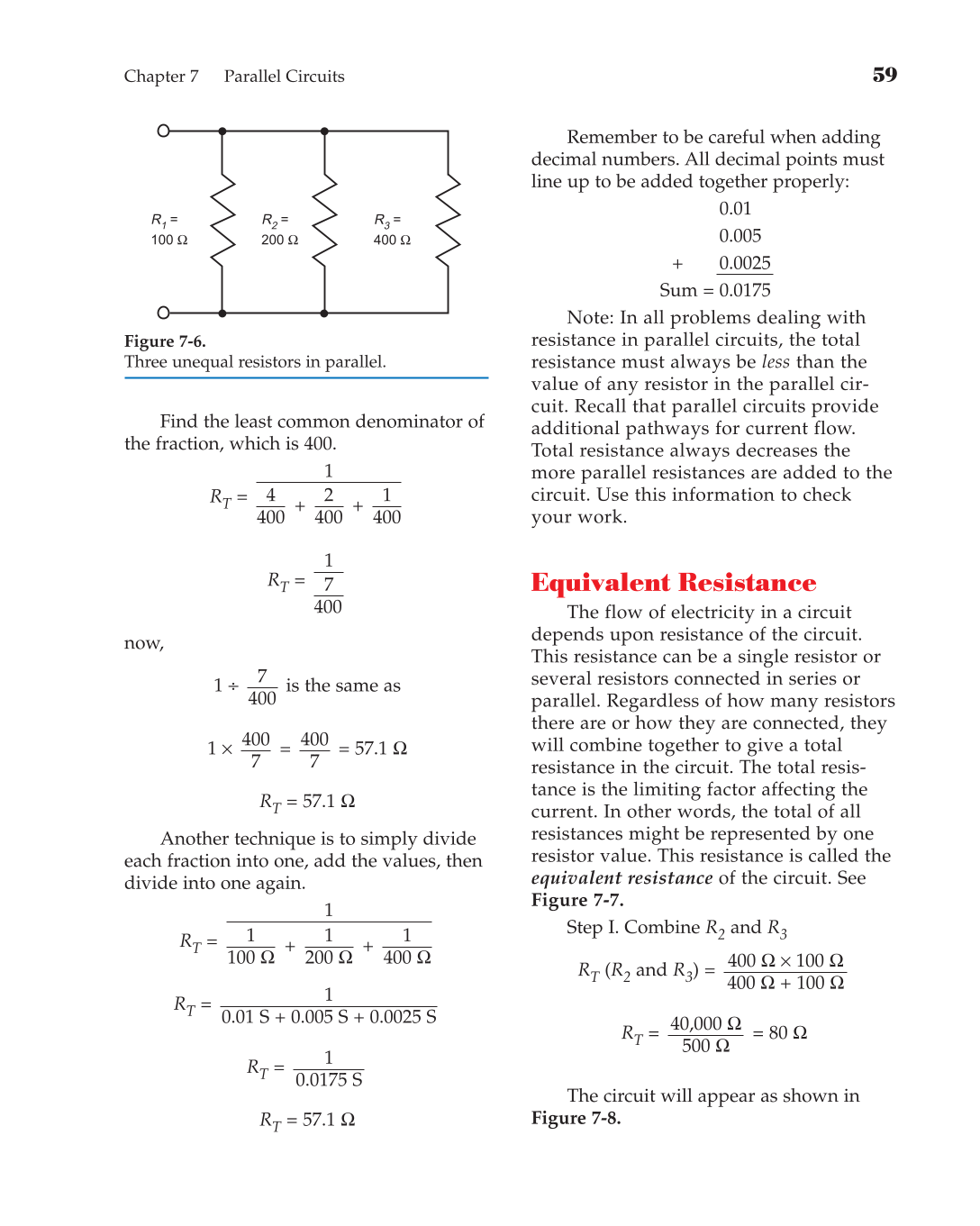
R3 =
400 Ω
R2 =
200 Ω
R1 =
100 Ω
Figure 7-6.
Three unequal resistors in parallel.
4
400
+
2
400
+
1
400
7
400
1
100 Ω
+
1
200 Ω
+
1
400 Ω