Step II. Combine the three resistors in
Figure 7-8. They are in series so:
R1 + (R2 and R3) + R4 =
100 Ω + 80 Ω + 500 Ω = 680 Ω
The circuit can now be represented by
Figure 7-9.
Electrically speaking, the circuits of
Figures 7-7, 7-8, and 7-9 are exactly the
same. RE (680 Ω) is the equivalent resistance
of the combination of R1, R2, R3, and R4.
Applications
Before leaving the study of series and
parallel circuits, let’s look at some familiar
applications.
A string of holiday or party lights
could be connected in a series or parallel
manner. Compare Figures 7-10 and 7-11.
The symbol is used for a lightbulb.
In Figure 7-10, the eight lightbulbs are
connected in series. All electrical current in
the circuit must pass through each lightbulb.
60
Electricity
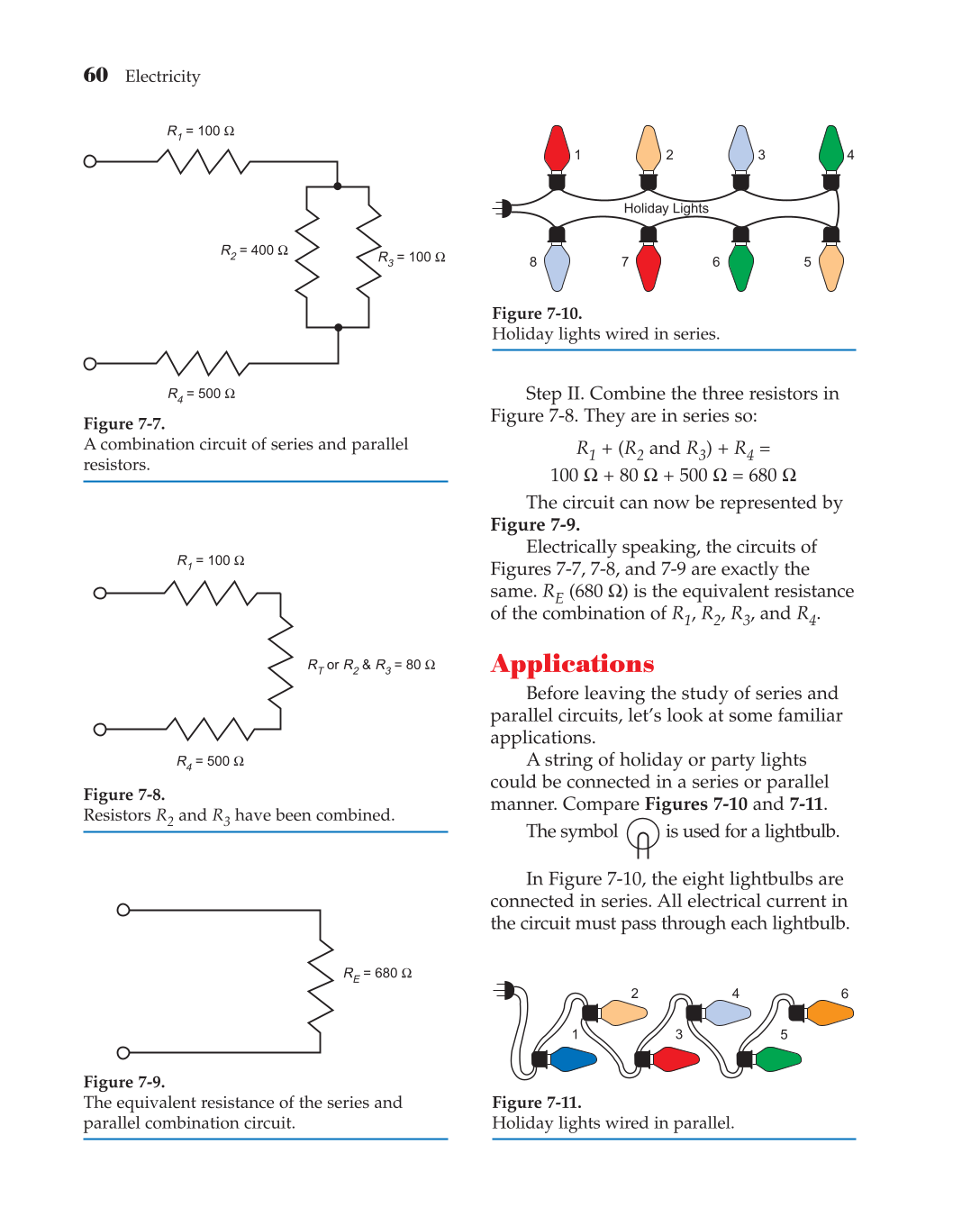
R3 = 100 Ω
R4 = 500 Ω
R1 = 100 Ω
R2 = 400 Ω
Figure 7-7.
A combination circuit of series and parallel
resistors.
R1 = 100 Ω
R4 = 500 Ω
RT or R2 & R3 = 80 Ω
Figure 7-8.
Resistors R2 and R3 have been combined.
RE = 680 Ω
Figure 7-9.
The equivalent resistance of the series and
parallel combination circuit.
1 2 3 4
5 6 7 8
Holiday Lights
Figure 7-10.
Holiday lights wired in series.
1
2
3
4
5
6
Figure 7-11.
Holiday lights wired in parallel.