Chapter 3 Introduction to Basic Electrical Circuit Materials 57
Conductor Resistance
Even though conductors provide a low-resistance
path for electron flow, they still have some resistance.
This resistance must be considered when long distances
are involved. There are four factors directly relating to the
resistance of conductors.
• Cross-sectional area of the conductor. The larger
the surface area or diameter of a conductor, the
lower the resistance.
• Type of conductor material. Different materials
have different resistance values.
• Length of conductor. The longer the conductor, the
greater the resistance.
• Temperature of material. Resistance of a material
rises with rising temperature.
Cross-sectional area of a conductor
Increasing the cross-sectional area of a conductor
increases the amount of current that can flow. To help
visualize this, use the flow of water through a pipe as an
example, Figure 3-6. A large-diameter pipe can carry
more gallons of water per minute than a small-diameter
pipe. An electric conductor operates in the same fashion.
The large-diameter conductor carries more electrons per
minute than a small-diameter conductor.
Length of conductor
The length of a conductor greatly affects its total
resistance. If one foot of wire has a certain resistance,
then ten feet of the same wire will have ten times more
resistance. Fifty feet of the wire will have fifty times
more resistance, and so on. As a conductor becomes
longer, it creates a voltage drop in a circuit. A circuit
using short lengths of wire, such as an electrical lab proj-
ect using three to six inches of No. 22 wire, does not cre-
ate a major problem. But long runs of wiring can create
electrical problems.
In Figure 3-8, a 10-amp load is connected to a cir-
cuit using No. 22 copper wire. The load is at a distance of
100 feet from the source. When the switch to the motor is
closed to connect the motor, the motor will heat up inter-
nally because an insufficient voltage is being applied. The
low voltage is a result of the voltage drop along the length
of the conductor. The loss of voltage can be computed
using Ohm’s law.
Figure 3-6. The larger the diameter of a pipe, the more
gallons flow per minute. The larger the diameter of a con-
ductor, the greater the current and the lower the resistance.
Type of material
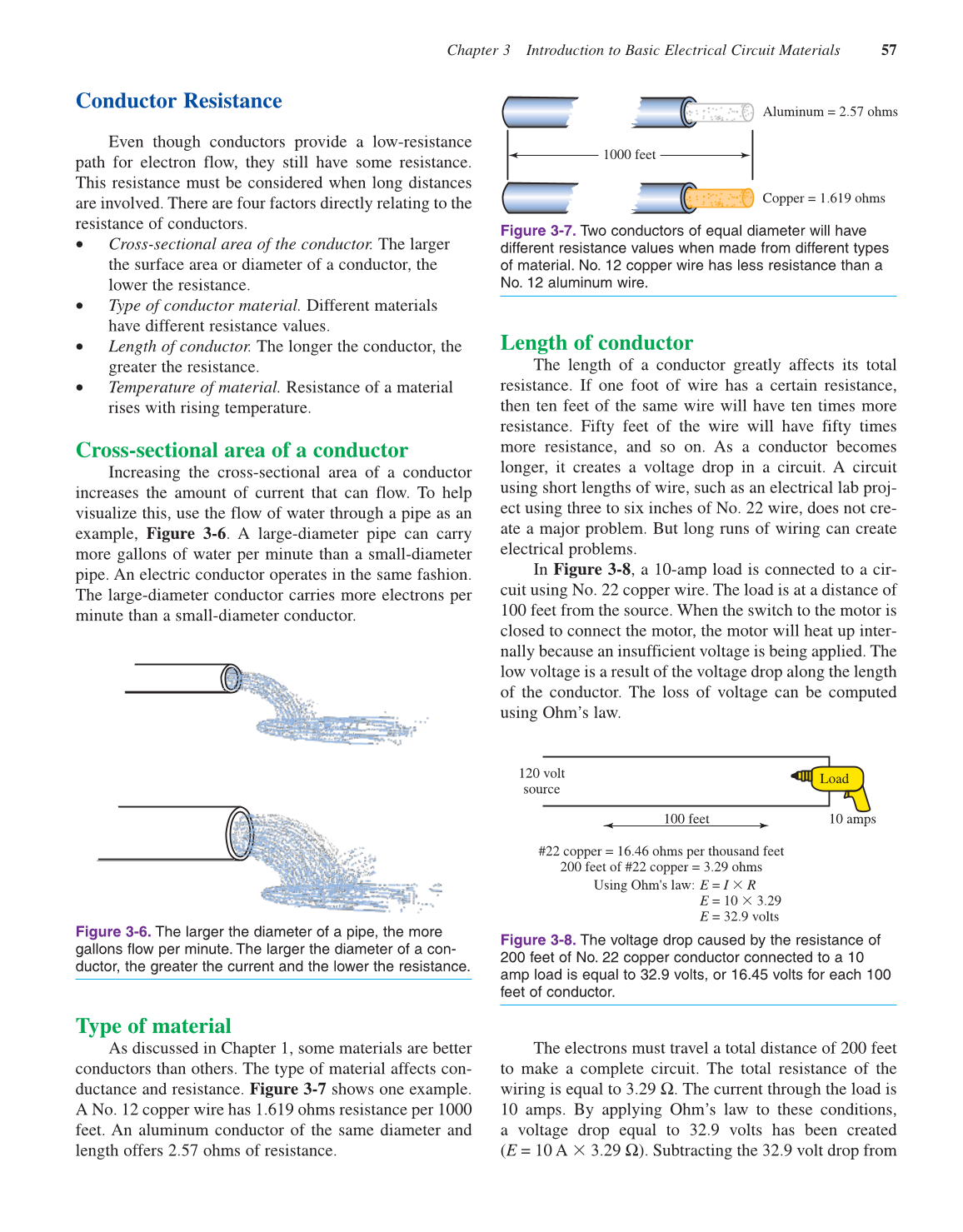
As discussed in Chapter 1, some materials are better
conductors than others. The type of material affects con-
ductance and resistance. Figure 3-7 shows one example.
A No. 12 copper wire has 1.619 ohms resistance per 1000
feet. An aluminum conductor of the same diameter and
length offers 2.57 ohms of resistance.
1000 feet
Aluminum = 2.57 ohms
Copper = 1.619 ohms
Figure 3-7. Two conductors of equal diameter will have
different resistance values when made from different types
of material. No. 12 copper wire has less resistance than a
No. 12 aluminum wire.
Load
10 amps
120 volt
source
100 feet
#22 copper = 16.46 ohms per thousand feet
200 feet of #22 copper = 3.29 ohms
Using Ohm's law: E = I R
E = 10 3.29
E = 32.9 volts
Figure 3-8. The voltage drop caused by the resistance of
200 feet of No. 22 copper conductor connected to a 10
amp load is equal to 32.9 volts, or 16.45 volts for each 100
feet of conductor.
The electrons must travel a total distance of 200 feet
to make a complete circuit. The total resistance of the
wiring is equal to 3.29 Ω. The current through the load is
10 amps. By applying Ohm’s law to these conditions,
a voltage drop equal to 32.9 volts has been created
(E = 10 A 3.29 Ω). Subtracting the 32.9 volt drop from