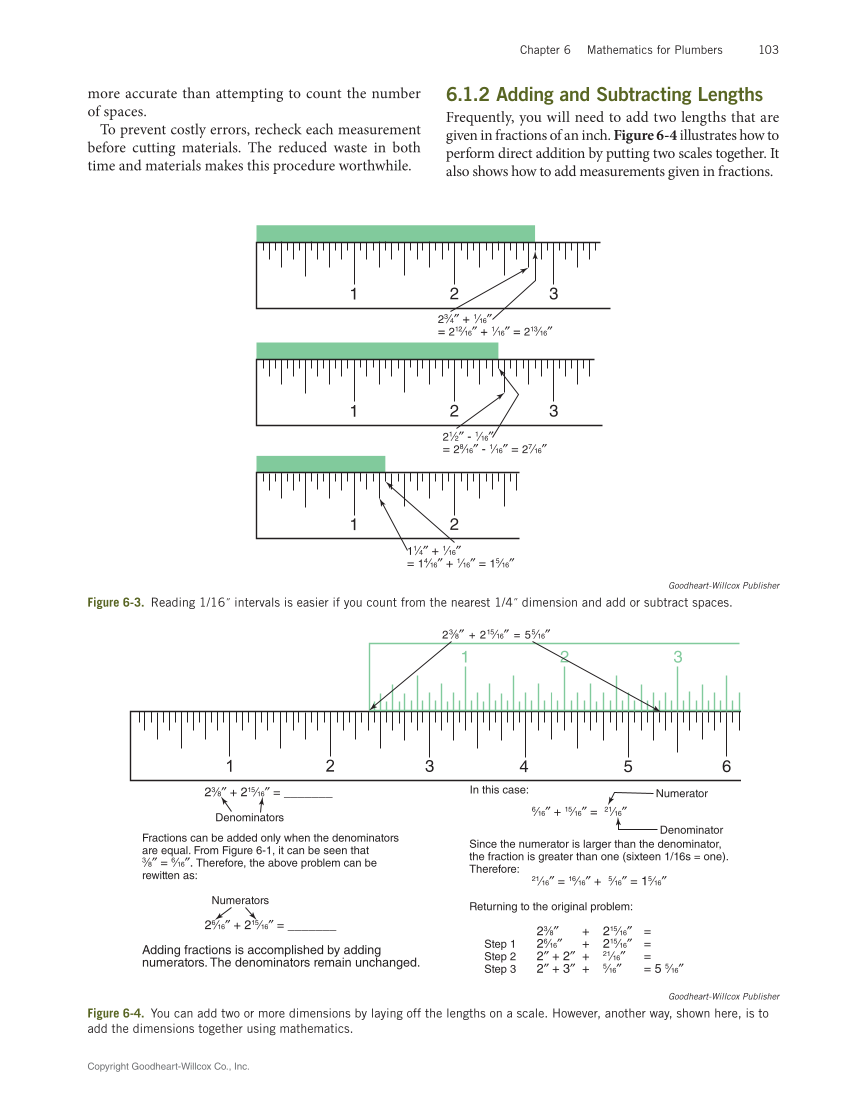
Copyright Goodheart-Willcox Co., Inc. Chapter 6 Mathematics for Plumbers 103 more accurate than attempting to count the number of spaces. To prevent costly errors, recheck each measurement before cutting materials. The reduced waste in both time and materials makes this procedure worthwhile. 6.1.2 Adding and Subtracting Lengths Frequently, you will need to add two lengths that are given in fractions of an inch. Figure 6-4 illustrates how to perform direct addition by putting two scales together. It also shows how to add measurements given in fractions. 1 2 3 1 2 1 2 3 23⁄4″ + 1⁄16″ = 212⁄16″ + 1⁄16″ = 213⁄16″ 21⁄2″ - 1⁄16″ = 28⁄16″ - 1⁄16″ = 27⁄16″ 11⁄4″ + 1⁄16″ = 14⁄16″ + 1⁄16″ = 15⁄16″ Goodheart-Willcox Publisher Figure 6-3. Reading 1/16˝ intervals is easier if you count from the nearest 1/4˝ dimension and add or subtract spaces. 1 2 3 4 5 6 23⁄8″ + 215⁄16″ = 55⁄16″ 23⁄8″ + 215⁄16″ = _______ Denominators Fractions can be added only when the denominators are equal. From Figure 6-1, it can be seen that 3⁄8″ = 6⁄16″. Therefore, the above problem can be rewitten as: Numerators 26⁄16″ + 215⁄16″ = _______ Adding fractions is accomplished by adding numerators. The denominators remain unchanged. 6⁄16″ + 15⁄16″ = 21⁄16″ Since the numerator is larger than the denominator, the fraction is greater than one (sixteen 1/16s = one). Therefore: 21⁄16″ = 16⁄16″ + 5⁄16″ = 15⁄16″ Returning to the original problem: 23⁄8″ + 215⁄16″ = Step 1 26⁄16″ + 215⁄16″ = Step 2 2″ + 2″ + 21⁄16″ = Step 3 2″ + 3″ + 5⁄16″ = 5 5⁄16″ Denominator Numerator In this case: 1 2 3 Goodheart-Willcox Publisher Figure 6-4. You can add two or more dimensions by laying off the lengths on a scale. However, another way, shown here, is to add the dimensions together using mathematics.