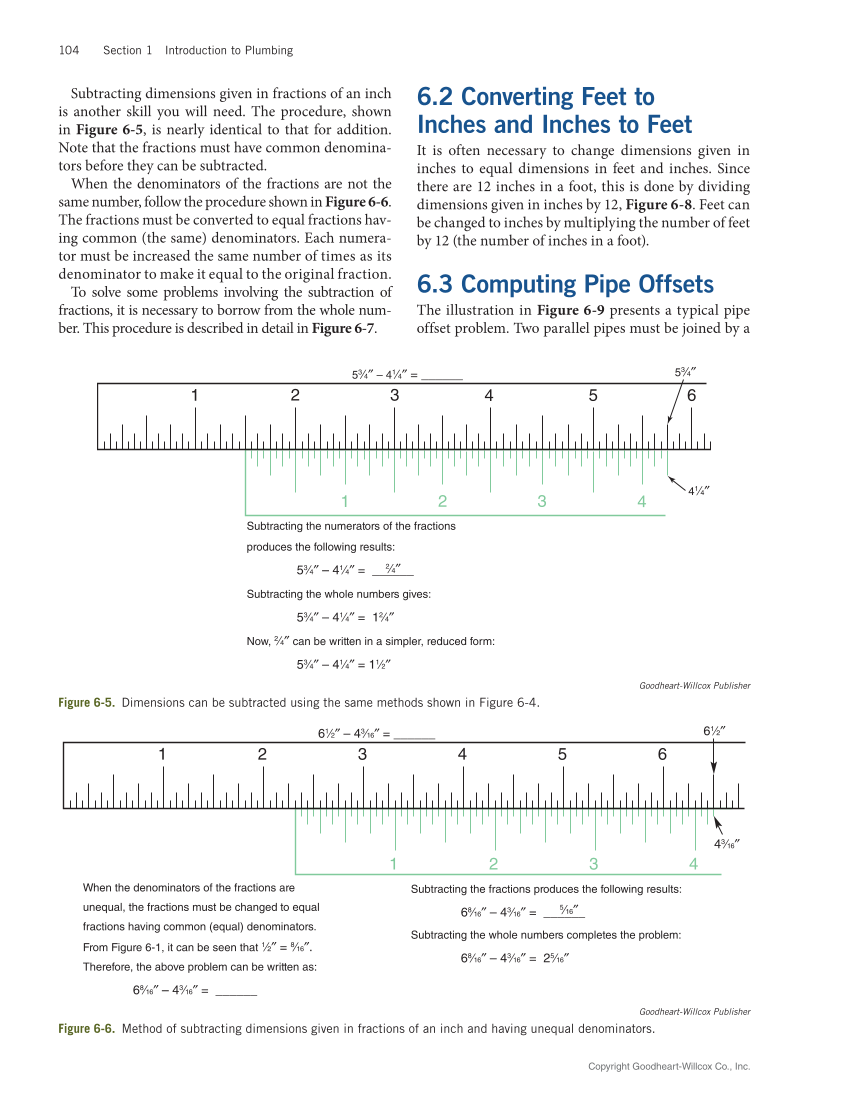
Copyright Goodheart-Willcox Co., Inc. 104 Section 1 Introduction to Plumbing Subtracting dimensions given in fractions of an inch is another skill you will need. The procedure, shown in Figure 6-5, is nearly identical to that for addition. Note that the fractions must have common denomina- tors before they can be subtracted. When the denominators of the fractions are not the same number, follow the procedure shown in Figure 6-6. The fractions must be converted to equal fractions hav- ing common (the same) denominators. Each numera- tor must be increased the same number of times as its denominator to make it equal to the original fraction. To solve some problems involving the subtraction of fractions, it is necessary to borrow from the whole num- ber. This procedure is described in detail in Figure 6-7. 6.2 Converting Feet to Inches and Inches to Feet It is often necessary to change dimensions given in inches to equal dimensions in feet and inches. Since there are 12 inches in a foot, this is done by dividing dimensions given in inches by 12, Figure 6-8. Feet can be changed to inches by multiplying the number of feet by 12 (the number of inches in a foot). 6.3 Computing Pipe Offsets The illustration in Figure 6-9 presents a typical pipe offset problem. Two parallel pipes must be joined by a 1 2 3 4 1 2 3 4 5 6 53⁄4″ – 41⁄4″ = ______ 41⁄4″ 53⁄4″ Subtracting the numerators of the fractions produces the following results: 53⁄4″ – 41⁄4″ = ______″4⁄2 Subtracting the whole numbers gives: 53⁄4″ – 41⁄4″ = 12⁄4″ Now, 2⁄4″ can be written in a simpler, reduced form: 53⁄4″ – 41⁄4″ = 11⁄2″ Goodheart-Willcox Publisher Figure 6-5. Dimensions can be subtracted using the same methods shown in Figure 6-4. 1 2 3 4 1 2 3 4 5 6 61⁄2″ – 43⁄16″ = ______ 61⁄2″ 43⁄16″ When the denominators of the fractions are unequal, the fractions must be changed to equal fractions having common (equal) denominators. From Figure 6-1, it can be seen that 1⁄2″ = 8⁄16″. Therefore, the above problem can be written as: 68⁄16″ – 43⁄16″ = ______ Subtracting the fractions produces the following results: 68⁄16″ – 43⁄16″ = ______″16⁄5 Subtracting the whole numbers completes the problem: 68⁄16″ – 43⁄16″ = 25⁄16″ Goodheart-Willcox Publisher Figure 6-6. Method of subtracting dimensions given in fractions of an inch and having unequal denominators.