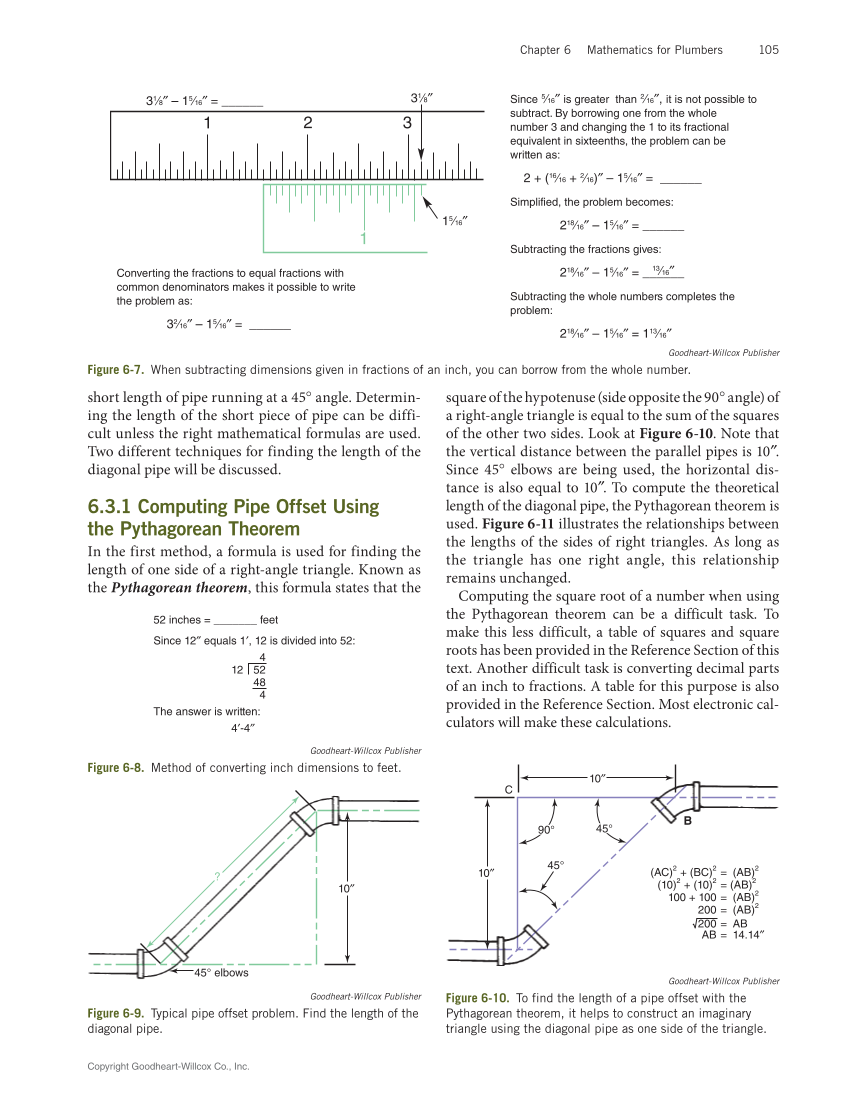
Copyright Goodheart-Willcox Co., Inc. Chapter 6 Mathematics for Plumbers 105 short length of pipe running at a 45° angle. Determin- ing the length of the short piece of pipe can be diffi- cult unless the right mathematical formulas are used. Two different techniques for finding the length of the diagonal pipe will be discussed. 6.3.1 Computing Pipe Offset Using the Pythagorean Theorem In the first method, a formula is used for finding the length of one side of a right-angle triangle. Known as the Pythagorean theorem, this formula states that the square of the hypotenuse (side opposite the 90° angle) of a right-angle triangle is equal to the sum of the squares of the other two sides. Look at Figure 6-10. Note that the vertical distance between the parallel pipes is 10″. Since 45° elbows are being used, the horizontal dis- tance is also equal to 10″. To compute the theoretical length of the diagonal pipe, the Pythagorean theorem is used. Figure 6-11 illustrates the relationships between the lengths of the sides of right triangles. As long as the triangle has one right angle, this relationship remains unchanged. Computing the square root of a number when using the Pythagorean theorem can be a difficult task. To make this less difficult, a table of squares and square roots has been provided in the Reference Section of this text. Another difficult task is converting decimal parts of an inch to fractions. A table for this purpose is also provided in the Reference Section. Most electronic cal- culators will make these calculations. 1 1 2 3 31⁄8″ – 15⁄16″ = ______ 15⁄16″ 31⁄8″ Converting the fractions to equal fractions with common denominators makes it possible to write the problem as: 32⁄16″ – 15⁄16″ = ______ Since 5⁄16″ is greater than 2⁄16″, it is not possible to subtract. By borrowing one from the whole number 3 and changing the 1 to its fractional equivalent in sixteenths, the problem can be written as: 2 + (16⁄16 + 2⁄16)″ – 15⁄16″ = ______ Simplified, the problem becomes: 218⁄16″ – 15⁄16″ = ______ Subtracting the fractions gives: 218⁄16″ – 15⁄16″ = ______″16⁄13 Subtracting the whole numbers completes the problem: 218⁄16″ – 15⁄16″ = 113⁄16″ Goodheart-Willcox Publisher Figure 6-7. When subtracting dimensions given in fractions of an inch, you can borrow from the whole number. 52 inches = _______ feet Since 12″ equals 1′, 12 is divided into 52: 4 12 52 48 4 The answer is written: 4′-4″ Goodheart-Willcox Publisher Figure 6-8. Method of converting inch dimensions to feet. 10″ ? 45° elbows Goodheart-Willcox Publisher Figure 6-9. Typical pipe offset problem. Find the length of the diagonal pipe. A (AC)2 + (BC)2 = (AB)2 (10)2 + (10)2 = (AB)2 100 + 100 = (AB)2 200 = (AB)2 √200 = AB AB = 14.14″ B 10″ 45° 10″ C 90° 45° Goodheart-Willcox Publisher Figure 6-10. To find the length of a pipe offset with the Pythagorean theorem, it helps to construct an imaginary triangle using the diagonal pipe as one side of the triangle.